Fractal Models for Quantifying Bow Speed and Contact Point
As a general principle of bowing, we know that as notes get higher, bow speed should increase or placement should move towards the bridge to maintain tone. As notes get lower, slowing the bow or moving away from the bridge is needed to keep tone consistent. However, the degree to which bow speed or placement should change is usually left to intuition. In fact, a proportional relationship exists between changes in frequency of vibration (pitch) and bow speed or placement.
Bow Speed
Bassist and acoustician Knut Guettler writes in his book, A Guide to Advanced Modern Double Bass Technique, “Speaking in physics, there is a proportional relationship between the frequency (pitch) of a note, and optimum bow speed, assuming constant pressure. As the frequency of the higher octave is twice that of the lower, it is in fact necessary for bow speed to be twice as fast on the upper note.” Although Guettler leaves it here, we can take this concept further.
When mapping out the ratios between frequency and bow speed, I noticed that we could use the framework from David Allen Moore’s Fractal Fingering as the basis for understanding these relationships and making them replicable at any scale. Fractal Fingering divides the string into six segments of equal length (on the G string: G-Bb, Bb-D, D-G, G-D, and D-D). When moving from the open G string to the Bb a minor third above it the string becomes 5/6ths of its original length and frequency of the Bb is 6/5ths of the frequency of the G as a result of the inverse relationship between string length and frequency. To maintain tone, the bow speed used to play the Bb must but 6/5ths of the bow speed used to play the G.
So what do we do with this information? My goal is not to have string players writing out equations for each change in pitch but rather to refine the intuition we necessarily rely on through the comprehension of these metrics gained by practicing changes in bow speed that are proportional to changes in pitch.
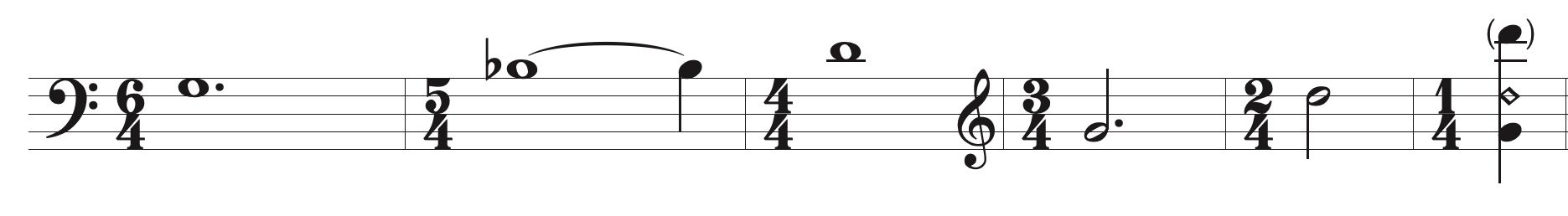
While it may seem initially confusing to increase bow speed by 6/5ths, we can measure this change in bow speed by maintaining the length of bow used and decreasing the amount of time allowed to travel that distance. For example, if the open G string is played using the entire bow over six beats, the Bb above it can be played using the entire bow over five beats, providing a simple way to measure that the bow speed has increased by 6/5ths.
Using the Fractal Fingering model, we can expand on this. As long as the entire bow is used for each note and contact point is consistent, the open G is played over six beats, the Bb above it will be played for 5 beats, the D above that for 4, the octave G harmonic for 3 beats, the D above that for 2, and the D above that will use the entire bow in one beat.
Contact Point
This same proportional relationship exists with respect to contact point. For each doubling of frequency, the bow should move halfway closer to the bridge (keep bow speed consistent). Beginning with a contact point near the fingerboard, divide the remaining length of string in to six equal portions and change the contact point by one segment for each of the pitches used in the bow speed exercise. As the contact point changes, the amount of hair, or tilt, of the bow should also be adjusted in proportion to the change in contact point. If full hair is used to play a given note, the octave about that should be played with half of the bow hair.
Fractals
These relationships are replicable at any scale. From any given pitch, ascending by a minor third will require a bow speed which is 6/5ths of the previous bow speed or a change of contact point 1/6th of the way closer to the bridge.
Exercises
The exercises below are a starting point for exploring these concepts. Of course, there are many other possible variations. These exercises are written in terms of bow speed, however, they can be easily adjusted for placement. In this case the time signature at the beginning of the exercise tells you how many portions to divide the distance between the contact point and the bridge into and the contact point will move one segment towards the bridge for each measure. In this case, each note will be played for the same duration. It is important to note that any extrapolation of contact point exercises beginning on a note on the extension will require that all notes in that exercise be played on the low C string. Because it is not the same length as the other strings, the proportions related to contact point are non-transferrable.